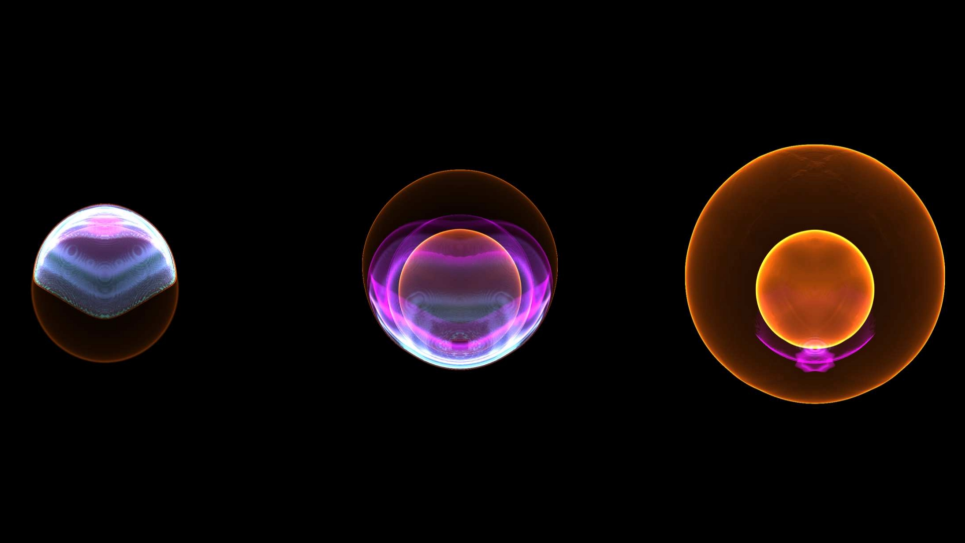
Distribution of the produced plasma on the QCD phase diagram in an event simulation. Each fluid cell is associated with a temperature and chemical potential, which is plotted here at an initial and later time. Knowledge of the EoS is essential in the complete T − µq range, with µq = 1/3µB.
This work aims to determine the properties of strongly interacting matter under extreme conditions from numerical simulations of the early universe, experimental heavy ion collisions, and compact stars.
This work aims to advance knowledge of the phase diagram and equation of state of strong interactions, by means of first-principle simulations. The researchers’ main goals are to locate the critical endpoint and explore the strongly interacting high-density regime relevant to neutron stars and their mergers—some of the main unsolved problems in the theory of strong interactions.
Ordinary hadronic matter undergoes a transition to a deconfined phase, quark-gluon plasma, at extremely high temperature or densities. In the universe, the reverse transition took place a few microseconds after the Big Bang: the basic building blocks of nature, the hadrons, were formed at this time. The Large Hadron Collider Heavy Ion program recreates this transition in the laboratory. Its DOE-funded detector upgrade will enable us to improve the precision in the data and thus search for new phenomena, such as experimental evidence for near criticality in the QCD transition. This project will compute experimental signatures to the onset of chiral critical behavior such as fluctuations of conserved charges.
A hydrodynamic description of the strongly interacting plasma requires the local equation of state at nonzero light and strange density. This project will compute it in the continuum limit with physical quark masses.
Brookhaven’s Relativistic Heavy Ion Collider explores the same transition in a broad range of densities, searching for a critical point in the QCD phase diagram—a difficult task, since there are no first principles to predict its location; direct simulations at finite density have remained elusive because of a sign problem. Thanks to a recent algorithmic development, these simulations, run with physical quark masses, can overcome this barrier and scan the phase diagram for features such as the cross-over line and the critical end point.
